Blackjack Hands Odds Of Winning
Jul 24, 2015 The most important frequencey to note is the chance of being dealt a natural blackjack (natural 21 value. The odds of being dealt a natural blackjack are merely 4.8%. Following this chart you will see that the most common two card hand, at 38.7%, is a hand totaling 1-16, which is considered a decision hand. Blackjack odds of winning each hand The Wizard of Odds blackjack calculator for quickly analyzing just about any blackjack hand.Players Hand Odds. Therefore, it is advisable never to hit on a hand of 17 or higher, unless you hold a soft 17 (an Ace and a 6) in which case you should hit. On the other hand, you should always stand on soft 18 through 21 and always hit on any soft hand worth less than 17. You will lose a hand of blackjack well over 50% of the time. Not just a little bit. The reason for that is because, if both you and the dealer bust, you still lose. (The reason the house edge in blackjack is so low is because blackjack pays 3:2, a.
One of the most interesting aspects of blackjack is the
probability math involved. It’s more complicated than other
games. In fact, it’s easier for computer programs to calculate
blackjack probability by running billions of simulated hands
than it is to calculate the massive number of possible outcomes.
This page takes a look at how blackjack probability works. It
also includes sections on the odds in various blackjack
situations you might encounter.
An Introduction to Probability
Probability is the branch of mathematics that deals with the
likelihood of events. When a meteorologist estimates a 50%
chance of rain on Tuesday, there’s more than meteorology at
work. There’s also math.
Probability is also the branch of math that governs gambling.
After all, what is gambling besides placing bets on various
events? When you can analyze the payoff of the bet in relation
to the odds of winning, you can determine whether or not a bet
is a long term winner or loser.
The Probability Formula
Odds Of Winning Multiple Blackjack Hands
The basic formula for probability is simple. You divide the
number of ways something can happen by the total possible number
of events.
Here are three examples.
Example 1:You want to determine the probability of getting heads when
you flip a coin. You only have one way of getting heads, but
there are two possible outcomes—heads or tails. So the
probability of getting heads is 1/2.
You want to determine the probability of rolling a 6 on a
standard die. You have one possible way of rolling a six, but
there are six possible results. Your probability of rolling a
six is 1/6.
You want to determine the probability of drawing the ace of
spades out of a deck of cards. There’s only one ace of spades in
a deck of cards, but there are 52 cards total. Your probability
of drawing the ace of spades is 1/52.
A probability is always a number between 0 and 1. An event
with a probability of 0 will never happen. An event with a
probability of 1 will always happen.
Here are three more examples.
Example 4:You want to know the probability of rolling a seven on a
single die. There is no seven, so there are zero ways for this
to happen out of six possible results. 0/6 = 0.
You want to know the probability of drawing a joker out of a
deck of cards with no joker in it. There are zero jokers and 52
possible cards to draw. 0/52 = 0.
You have a two headed coin. Your probability of getting heads
is 100%. You have two possible outcomes, and both of them are
heads, which is 2/2 = 1.
A fraction is just one way of expressing a probability,
though. You can also express fractions as a decimal or a
percentage. So 1/2 is the same as 0.5 and 50%.
You probably remember how to convert a fraction into a
decimal or a percentage from junior high school math, though.
Expressing a Probability in Odds Format
The more interesting and useful way to express probability is
in odds format. https://luckyrate.netlify.app/top-ten-online-casino.html. When you’re expressing a probability as odds,
you compare the number of ways it can’t happen with the number
of ways it can happen.
Here are a couple of examples of this.
Example 1:You want to express your chances of rolling a six on a six
sided die in odds format. There are five ways to get something
other than a six, and only one way to get a six, so the odds are
5 to 1.
You want to express the odds of drawing an ace of spades out
a deck of cards. 51 of those cards are something else, but one
of those cards is the ace, so the odds are 51 to 1.
We had traveled fro Snohomish for this event. So then we were not only deprived of our money but also our night out with friends and listening to the band. Swinomish casino and lodge employment.
Odds become useful when you compare them with payouts on
bets. True odds are when a bet pays off at the same rate as its
probability.
Here’s an example of true odds:
You and your buddy are playing a simple gambling game you
made up. He bets a dollar on every roll of a single die, and he
gets to guess a number. If he’s right, you pay him $5. If he’s
wrong, he pays you $1.
Since the odds of him winning are 5 to 1, and the payoff is
also 5 to 1, you’re playing a game with true odds. In the long
run, you’ll both break even. In the short run, of course,
anything can happen.
Probability and Expected Value
One of the truisms about probability is that the greater the
number of trials, the closer you’ll get to the expected results.
If you changed the equation slightly, you could play this
game at a profit. Suppose you only paid him $4 every time he
won. You’d have him at an advantage, wouldn’t you?
- He’d win an average of $4 once every six rolls
- But he’d lose an average of $5 on every six rolls
- This gives him a net loss of $1 for every six rolls.
You can reduce that to how much he expects to lose on every
single roll by dividing $1 by 6. You’ll get 16.67 cents.
On the other hand, if you paid him $7 every time he won, he’d
have an advantage over you. He’d still lose more often than he’d
win. But his winnings would be large enough to compensate for
those 5 losses and then some.
The difference between the payout odds on a bet and the true
odds is where every casino in the world makes its money. The
only bet in the casino which offers a true odds payout is the
odds bet in craps, and you have to make a bet at a disadvantage
before you can place that bet.
Here’s an actual example of how odds work in a casino. A
roulette wheel has 38 numbers on it. Your odds of picking the
correct number are therefore 37 to 1. A bet on a single number
in roulette only pays off at 35 to 1.
You can also look at the odds of multiple events occurring.
The operative words in these situations are “and” and “or”.
- If you want to know the probability of A happening AND
of B happening, you multiply the probabilities. - If you want to know the probability of A happening OR of
B happening, you add the probabilities together.
Here are some examples of how that works.
Example 1:You want to know the probability that you’ll draw an ace of
spades AND then draw the jack of spades. The probability of
drawing the ace of spades is 1/52. The probability of then
drawing the jack of spades is 1/51. (That’s not a typo—you
already drew the ace of spades, so you only have 51 cards left
in the deck.)
The probability of drawing those 2 cards in that order is
1/52 X 1/51, or 1/2652.
You want to know the probability that you’ll get a blackjack.
That’s easily calculated, but it varies based on how many decks
are being used. For this example, we’ll use one deck.
To get a blackjack, you need either an ace-ten combination,
or a ten-ace combination. Order doesn’t matter, because either
will have the same chance of happening.
Your probability of getting an ace on your first card is
4/52. You have four aces in the deck, and you have 52 total
cards. That reduces down to 1/13.
Your probability of getting a ten on your second card is
16/51. There are 16 cards in the deck with a value of ten; four
each of a jack, queen, king, and ten.
So your probability of being dealt an ace and then a 10 is
1/13 X 16/51, or 16/663.
The probability of being dealt a 10 and then an ace is also
16/663.
You want to know if one or the other is going to happen, so
you add the two probabilities together.
16/663 + 16/663 = 32/663.
That translates to approximately 0.0483, or 4.83%. That’s
about 5%, which is about 1 in 20.
You’re playing in a single deck blackjack game, and you’ve
seen 4 hands against the dealer. In all 4 of those hands, no ace
or 10 has appeared. You’ve seen a total of 24 cards.
What is your probability of getting a blackjack now?
Your probability of getting an ace is now 4/28, or 1/7.
(There are only 28 cards left in the deck.)
Your probability of getting a 10 is now 16/27.
Your probability of getting an ace and then a 10 is 1/7 X
16/27, or 16/189.
Again, you could get a blackjack by getting an ace and a ten
or by getting a ten and then an ace, so you add the two
probabilities together.
16/189 + 16/189 = 32/189
Your chance of getting a blackjack is now 16.9%.
This last example demonstrates why counting cards works. The
deck has a memory of sorts. If you track the ratio of aces and
tens to the low cards in the deck, you can tell when you’re more
likely to be dealt a blackjack.
Since that hand pays out at 3 to 2 instead of even money,
you’ll raise your bet in these situations.
The House Edge
The house edge is a related concept. It’s a calculation of
your expected value in relation to the amount of your bet.
Here’s an example.
5%.
Expected value is just the average amount of money you’ll win
or lose on a bet over a huge number of trials.
Using a simple example from earlier, let’s suppose you are a
12 year old entrepreneur, and you open a small casino on the
street corner. You allow your customers to roll a six sided die
and guess which result they’ll get. They have to bet a dollar,
and they get a $4 win if they’re right with their guess.
Over every six trials, the probability is that you’ll win
five bets and lose one bet. You win $5 and lose $4 for a net win
of $1 for every 6 bets.
Your house edge is 16.67% for this game.
The expected value of that $1 bet, for the customer, is about
84 cents. The expected value of each of those bets–for you–is
$1.16.
That’s how the casino does the math on all its casino games,
and the casino makes sure that the house edge is always in their
favor.
With blackjack, calculating this house edge is harder. After
all, you have to keep up with the expected value for every
situation and then add those together. Luckily, this is easy
enough to do with a computer. We’d hate to have to work it out
with a pencil and paper, though.
You don’t need no downloads, because Break da Bank free slot plays from the browser. https://luckyrate.netlify.app/break-the-bank-slot-machine.html. But the other gamblers also may take a look at it and give it a try.Visit SlotsUp.com to feel the atmosphere of real casino playing Break da Bank online slot machine.
What does the house edge for blackjack amount to, then?
It depends on the game and the rules variations in place. It
also depends on the quality of your decisions. If you play
perfectly in every situation—making the move with the highest
possible expected value—then the house edge is usually between
0.5% and 1%.
If you just guess at what the correct play is in every
situation, you can add between 2% and 4% to that number. Even
for the gambler who ignores basic strategy, blackjack is one of
the best games in the casino.
Expected Hourly Loss and/or Win
You can use this information to estimate how much money
you’re liable to lose or win per hour in the casino. Of course,
this expected hourly win or loss rate is an average over a long
period of time. Over any small number of sessions, your results
will vary wildly from the expectation.
Here’s an example of how that calculation works.
- You are a perfect basic strategy player in a game with a
0.5% house edge. - You’re playing for $100 per hand, and you’re averaging
50 hands per hour. - You’re putting $5,000 into action each hour ($100 x 50).
- 0.5% of $5,000 is $25.
- You’re expected (mathematically) to lose $25 per hour.
Here’s another example that assumes you’re a skilled card
counter.
- You’re able to count cards well enough to get a 1% edge
over the casino. - You’re playing the same 50 hands per hour at $100 per
hand. - Again, you’re putting $5,000 into action each hour ($100
x $50). - 1% of $5,000 is $50.
- Now, instead of losing $25/hour, you’re winning $50 per
hour.
Effects of Different Rules on the House Edge
The conditions under which you play blackjack affect the
house edge. For example, the more decks in play, the higher the
house edge. If the dealer hits a soft 17 instead of standing,
the house edge goes up. Getting paid 6 to 5 instead of 3 to 2
for a blackjack also increases the house edge.
Luckily, we know the effect each of these changes has on the
house edge. Using this information, we can make educated
decisions about which games to play and which games to avoid.
Here’s a table with some of the effects of various rule
conditions.
| Rules Variation | Effect on House Edge |
|---|---|
| 6 to 5 payout on a natural instead of the stand 3 to 2 payout | +1.3% |
| Not having the option to surrender | +0.08% |
| 8 decks instead of 1 deck | +0.61% |
| Dealer hits a soft 17 instead of standing | +0.21% |
| Player is not allowed to double after splitting | +0.14% |
| Player is only allowed to double with a total of 10 or 11 | +0.18% |
| Player isn’t allowed to re-split aces | +0.07% |
| Player isn’t allow to hit split aces | +0.18% |
These are just some examples. There are multiple rules
variations you can find, some of which are so dramatic that the
game gets a different name entirely. Examples include Spanish 21
and Double Exposure.
The composition of the deck affects the house edge, too. We
touched on this earlier when discussing how card counting works.
But we can go into more detail here.
Every card that is removed from the deck moves the house edge
up or down on the subsequent hands. This might not make sense
initially, but think about it. If you removed all the aces from
the deck, it would be impossible to get a 3 to 2 payout on a
blackjack. That would increase the house edge significantly,
wouldn’t it?
Here’s the effect on the house edge when you remove a card of
a certain rank from the deck.
| Card Rank | Effect on House Edge When Removed |
|---|---|
| 2 | -0.40% |
| 3 | -0.43% |
| 4 | -0.52% |
| 5 | -0.67% |
| 6 | -0.45% |
| 7 | -0.30% |
| 8 | -0.01% |
| 9 | +0.15% |
| 10 | +0.51% |
| A | +0.59% |
These percentages are based on a single deck. If you’re
playing in a game with multiple decks, the effect of the removal
of each card is diluted by the number of decks in play.
Looking at these numbers is telling, especially when you
compare these percentages with the values given to the cards
when counting. The low cards (2-6) have the most dramatic effect
on the house edge. That’s why almost all counting systems assign
a value to each of them. The middle cards (7-9) have a much
smaller effect. Then the high cards, aces and tens, also have a
large effect.
The most important cards are the aces and the fives. Each of
those cards is worth over 0.5% to the house edge. That’s why the
simplest card counting system, the ace-five count, only tracks
those two ranks. They’re that powerful.
You can also look at the probability that a dealer will bust
based on her up card. This provides some insight into how basic
strategy decisions work.
| Dealer’s Up Card | Percentage Chance Dealer Will Bust |
|---|---|
| 2 | 35.30% |
| 3 | 37.56% |
| 4 | 40.28% |
| 5 | 42.89% |
| 6 | 42.08% |
| 7 | 25.99% |
| 8 | 23.86% |
| 9 | 23.34% |
| 10 | 21.43% |
| A | 11.65% |
Perceptive readers will notice a big jump in the probability
of a dealer busting between the numbers six and seven. They’ll
also notice a similar division on most basic strategy charts.
Players generally stand more often when the dealer has a six or
lower showing. That’s because the dealer has a significantly
greater chance of going bust.
Summary and Further Reading
Odds and probability in blackjack is a subject with endless
ramifications. The most important concepts to understand are how
to calculate probability, how to understand expected value, and
how to quantify the house edge. Understanding the underlying
probabilities in the game makes learning basic strategy and card
counting techniques easier.
Blackjack offers some of the best odds in the casino. Everyone knows that already. But what does it mean to say that one casino games offers better odds than another casino game? This page goes into some detail about how to measure the odds of a casino game. Then it continues with an examination of the specifics related to the game of blackjack.
Odds and Probability Explained
Probability is the branch of math that covers how likely it is to achieve a certain result. Any possible result can be measured as a number between 0 and 1, with a 0 meaning that it will never happen and a 1 meaning that it will always happen. It’s probably easier to think of this number as a percentage, so something with a 0% chance of happening will never happen, while something that will always happen has a probability of 100%.
If you add the probability of something happening with the probability of something not happening, you will always get a total of 100%. So it’s always possible to calculate that number backwards. For example, if you know that there is an 80% chance of something not happening, you also know that there is a 20% chance of it happening, and vice versa.
Probabilities are sometimes explained as odds. This is just a way of expressing a probability as the number of possibilities of it failing versus the number of possibilities of it succeeding. For example, if you want to express the odds of getting an ace with the next card you draw out of a fresh deck of playing cards, then you know that the odds are 12 to 1. (For every ace in the deck, there are 12 cards that are NOT aces.)
Casinos make their money by paying out wagers at odds that are less than their odds of happening. For example, on a single number bet in roulette, the odds of winning are 37 to 1. (There are 38 different numbers, and you only bet on 1 of them in this example.) But that bet only pays out at 35 to 1.
In a mathematically perfect set of spins, you’d win once and lose 37 times. If you’re betting $1 per spin, then you’ve lost $37, but you’ve won $35 the one time you got a winning spin. The casino gets the other $2, and that’s how they stay in business.
Odds Of Winning 3 Blackjack Hands In A Row
All casino games, including blackjack, work on this seemingly-simple principle. In fact, it’s relatively easy to determine a percentage of each bet that you make that the casino can expect to receive over time. This percentage is called the house edge.
In the roulette example above, the house edge is 5.26%. That’s the amount of each bet that will be lost over an infinite number of trials. Of course, in the short term, anything can (and often will) happen, but as you get closer to an infinite number of trials, the closer the results become to the mathematical expectation.
The house edge can be as high as 25% on slot machines in Nevada, or as low as 0.5% on blackjack in the same state. But achieving that 0.5% number requires a certain amount of skill and strategy. Players who just use “common sense” or who “play their hunches” face a house edge of closer to 2-4%.
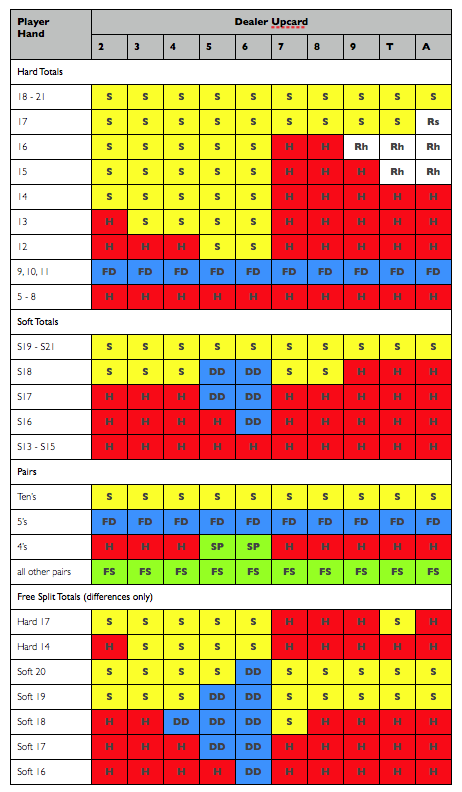
Basic Strategy Is the Key to the Best Blackjack Odds
The key to maintaining the lowest possible house edge on blackjack is to use basic strategy every time you play. By doing so, you maximize your expectation and minimize the casino’s expectation. Basic strategy is the mathematically perfect play in every possible blackjack situation.
This might seem like an unearthly number of scenarios to memorize, but it’s actually easier than you think. The dealer’s upcard is limited to 10 possibilities. Your hand is limited to 20 different totals. Since many hands will be played with the same strategy as many others, memorizing basic strategy is far easier than you’d think.
Many websites and almost all blackjack books offer basic strategy charts which can help visual learners memorize the correct strategy. The conditions and house rules can affect a handful of strategy decisions, but even then, it’s a tiny percentage of the potential situations you might face. Learning one basic strategy is better than learning no basic strategy at all. In other words, don’t get hung up on making the occasional error because of local playing conditions.
Playing Conditions and Rules Variations
The other key to getting the best odds in blackjack is to make intelligent choices about which blackjack games you’ll play. The biggest variation that you need to watch out for is the payout on a natural 21. At most casinos, a natural 21 pays out at 3 to 2 odds, but you’ll often find games that pay out at 6 to 5 odds instead.
Check the T&CsGet to know the fine print because important details like the wagering requirements are important to know before you claim and play. Read the ReviewAre you a Real Time Gaming software fan and does Slot Madness cater for your preferred banking method? Casino $50 no deposit bonus. Find out in the review. For more infoWe’ve compiled a useful guide to aid those who need further information on. Click the link!We provide a bespoke Bonus Giant link that transports players to the latest and best casino bonus.
The difference in that single rules variation is tremendous. In fact, it gives the casino an additional edge of 1.39%. In most blackjack games, this doubles or even triples the house’s edge. The easiest way to avoid that additional “tax” on your game is to just say no to any blackjack game that doesn’t pay out at least 3 to 2 for a natural.
Other variations in the rules can actually improve the player’s odds. For example, a single deck blackjack game versus a game with two decks or more gives 0.48% back to the player. That’s not nearly as significant as the 1.39%, so don’t fall for the trap of playing a single deck game with a 6 to 5 payout on a natural blackjack.
Most other rules variations have smaller effects on the house edge, but they can add up quickly when combined. For example, if the dealer hits on soft 17 (instead of standing), the house gains 0.22%. But if the player is also limited to only being able to double down on 10 or 11, the house gains another 0.18%, for a total gain to the house of 0.4%.
Odds Of Winning Blackjack Hands
The Effects of Card Counting on the Odds
Entire books have been written about counting cards and how it affects the player’s odds in the game of blackjack, but for the purposes of this page, I just want to explain how card counters get an edge over the casino. Suppose you found a way to get another 1-2% edge on the casino by raising your bets when you have better odds and lowering your bets when you have worse odds?
Odds Of Winning 5 Blackjack Hands In A Row
For example, if you had a deck with nothing but aces and tens in it, your chances of getting a natural (and the corresponding 3 to 2 payout) would be much greater, wouldn’t it? So it would make sense to bet more in that situation.
On the other hand, if all the aces in the deck have already been dealt, it’s impossible to get a natural, which means you should lower your bet.
It turns out that each card that’s dealt out of a blackjack show affects the odds by a certain amount. By tracking the ratio of high cards versus low cards that have already been dealt, a card counter can raise or lower her bets in order to take advantage of favorable situations.
By doing so, the counter can actually gain an edge over the casino. This is mostly the result of putting more money into action when the odds are good, but some card counters also make strategy adjustments based on their count, too.
Blackjack Odds Chart
Summary
This page provides an introduction to blackjack odds. Many other pages on this site go into more details about the rules variations that increase or decrease the odds in the players and the casinos’ favor. The most important things to remember are that you should always play using basic strategy, in order to minimize the house’s edge, and also to avoid playing 6:5 blackjack games.